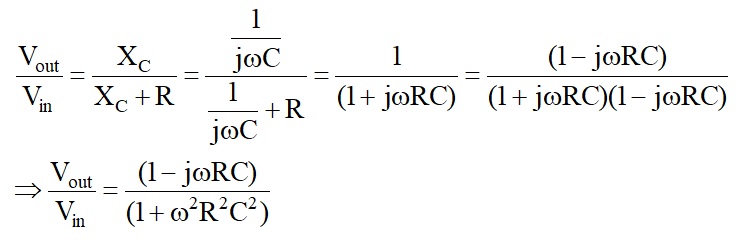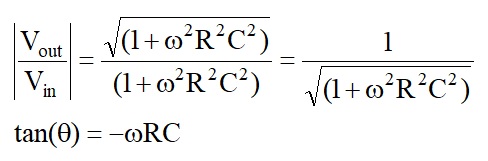www.ikes.16mb.com

Version 220319

Capacitors in AC circuits

Introduction to the sine wave formula



An alternating current can be represented by

I = I0sin(ωt),

where I0 is the amplitude of the current and ω = 2π×frequency

For an ideal resistor, using Ohm's Law, the alternating voltage across a resistor, R, is give by

V = R×I0sin(ωt),

As can be seen from the current and voltage formulae, they are always in phase.

For a capacitor, the voltage across the capacitor is given by the charge, Q, divided by the capacitance, C,   => V = Q/C

The charge is given by Q = ∫I.dt

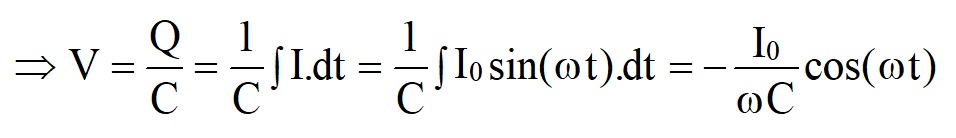
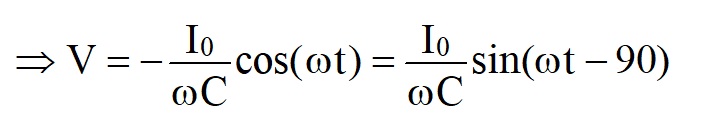
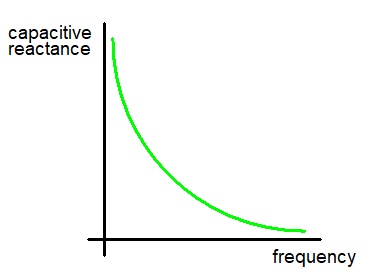
This expression shows that the applied voltage across a capacitor lags the current by 90°.

For an ideal capacitor, the ratio of the applied voltage to the current passing through is called the reactance, Xc.
This should be compared to Ohm's law where the ratio of voltage to current is called resistance.

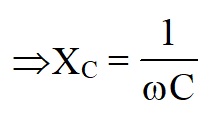
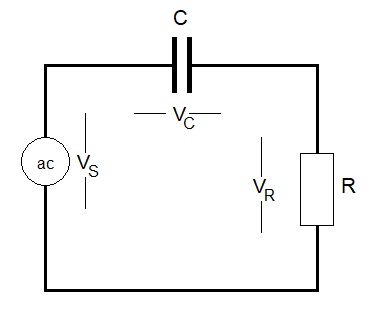
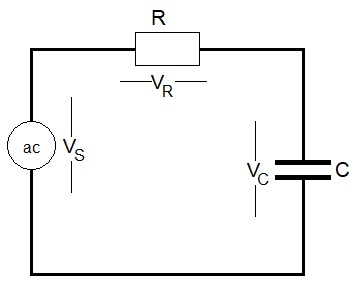
As can be seen from the formula, capacitive reactance decreases as frequency increases.


A capacitor and resistor in series.

The current passing in the circuit is

I = I0sin(ωt),

and is the same through both the resistor and capacitor as they are in series.

=> The voltage across the resistor, R, is

VR = RI0sin(ωt),

and is in phase with the current.
=> The voltage across the capacitor, C, is

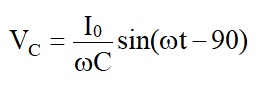
=> The voltage across the capacitor lags the current by 90° (π/2 radians)

To make sense of what this means, it is helpful to represent these voltages and currents on a diagram.

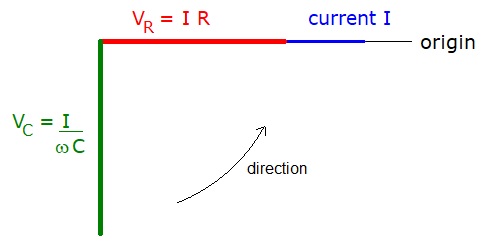
Kirchhoff's 2nd Law states that the algebraic sum of the potential differences in any loop must equal zero. (Conservation of energy)
Since VC and VR are not in phase they must be added as vectors

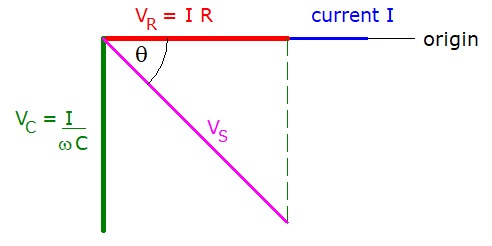
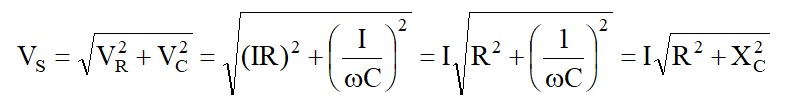
Looking at the final term and comparing to Ohm's Law, √(R2 + XC2) represents the opposition to the current from the power supply.
Containing both resistance and reactance, it is called the Impedance of the circuit with the symbol Z.

Z = √(R2 + XC2)

The power supply voltage is out of phase with the current by angle θ, where tanθ = VC/VR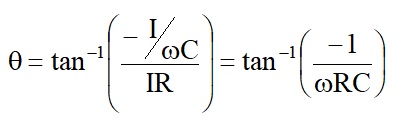

The angle θ is often referred to as the phase angle.

RC Potential Divider

Looking at this circuit again and using the Potential Divider formula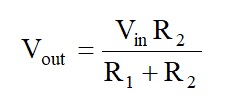

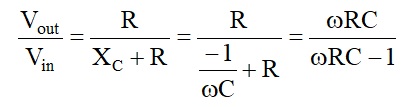
If VS is the input voltage (Vin) and VR is the output (Vout)

=> when ω is small,   Vout/Vin ≈ ωRC and

when ω is large,   Vout/Vin ≈ 1

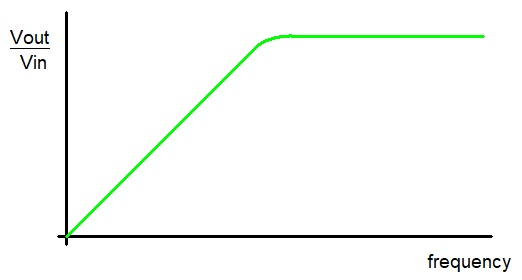
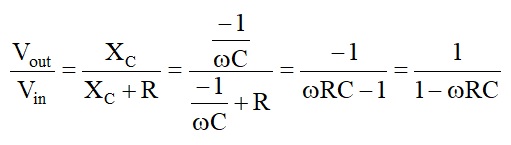
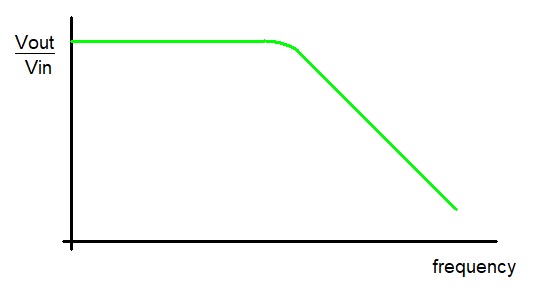
This circuit works as a simple 'High Pass filter' - it attenuates low frequencies but passes high frequencies.

Swapping R and C around so that VS is the input voltage (Vin) and VC is the output (Vout) gives

=> when ω is small,   Vout/Vin ≈ 1 and

when ω is large,   Vout/Vin ≈ (-)1/ωRC

This circuit works as a simple 'Low Pass filter' - it attenuates high frequencies but passes low frequencies.

All of the above analysis is only approximate because the phase relationship between Vout and Vin has been ignored.

AC Circuits in Complex Form

It is very convenient to use Complex numbers for processing AC calculations.

The real axis represents Resistance.   Power is only dissipated in pure (real) resistance.

The imaginary axis represents Reactance.   Power is NOT dissipated in reactance (averaged over a time period).

Unfortunately, i is the symbol used to represent current.
To avoid confusion in circuit calculations, j is used to represent √(-1)

Capacitive reactance, XC, becomes -j/ωC
Multiplying top and bottom by j gives XC = 1/jωC

Analysing this circuit again